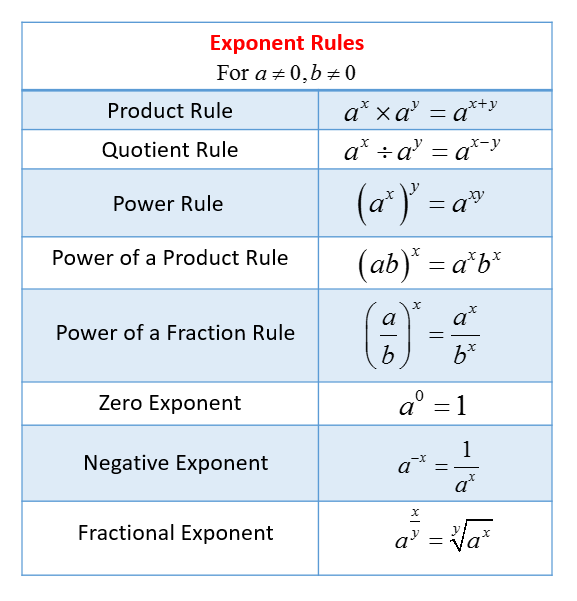
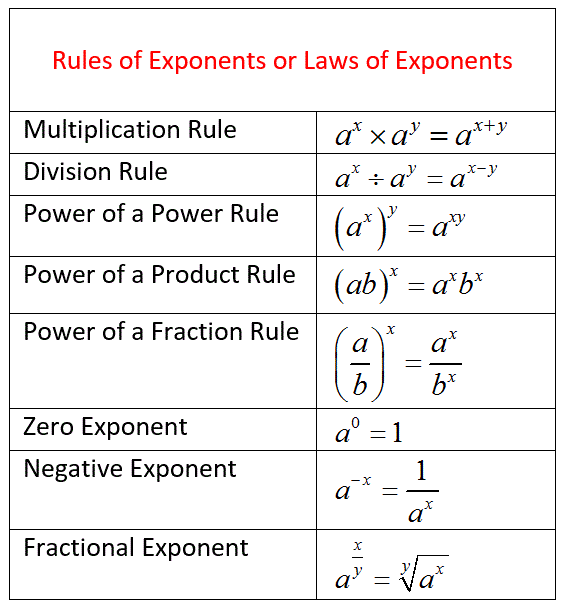
Rules Of Exponents Chart
Rules Of Exponents Chart - 2^3 ⋅ 2^4 = 2^ (3+4) = 2^7 = 128. A 0 = 1 2. Web each exponent rules chart on this page summarizes how to use the power rule, fraction rule, product rule, the negative rule, log to exponents and more! Web there are several laws of exponents (sometimes called exponent laws or rules of exponents), but this page will cover product rule, quotient rule, and negative exponent rule. ( xy )m = x m ym. When dividing two quantities with the same base, subtract exponents: Web rules of exponents 1. The base a raised to the power of n is equal to the multiplication of a, n times: X (1 n) = n√x. The exponent laws are the tools needed for working with expressions involving exponents. The laws of exponents illustrate how to simplify numbers using the properties of. Putting all the rules together, we can simplify more complex expression containing exponents. 2^3 ⋅ 2^4 = 2^ (3+4) = 2^7 = 128. A n = a × a ×. \large \displaystyle x^0 = 1 x0 = 1, for x = \not 0 x = 0. A fractional exponent like 1/n means to take the nth root: When multiplying two quantities with the same base, add exponents: Web rules of exponents 1. Web properties of exponents. 3 ) 2 = (2•2•2)(2•2•2) = 2. Putting all the rules together, we can simplify more complex expression containing exponents. A is the base and n is the exponent. A negative exponent tells you that the factor is on the wrong side of the fraction bar. The base a raised to the power of n is equal to the multiplication of a, n times: 2^3 ⋅ 2^4. All of the rules for manipulating exponents may be deduced from the laws of multiplication and division that you are already familiar with. A 0 = 1 2. \large\displaystyle x^m \cdot x^n = x^ {m+n} xm ⋅ xn = xm+n. Web rules, formulas and practice problems. 3 ) 2 = 2. A n am an m 3. Web the exponent (the number 2) is the number of bases (the number 5) you multiply together. 2^3 ⋅ 2^4 = 2^ (3+4) = 2^7 = 128. A negative exponent tells you that the factor is on the wrong side of the fraction bar. When exponents are identical but bases differ: Web rules, formulas and practice problems. $$ \boxed{ x^a \cdot x^ b = x^{a \red + b} } \\ \text{example : For all real numbers x and y and real number constants m and n. 2^3 ⋅ 2^4 = 2^ (3+4) = 2^7 = 128. A n = a × a ×. When a base to an exponent is raised to another exponent, keep the base the same and multiply the exponents: Web the rules of exponents allow you to simplify expressions involving exponents. The laws of exponents illustrate how to simplify numbers using the properties of. When dividing two quantities with the same base, subtract exponents: Web in this article, we. Web the rules of exponents allow you to simplify expressions involving exponents. The base a raised to the power of n is equal to the multiplication of a, n times: $$ \boxed{ x^a \cdot x^ b = x^{a \red + b} } \\ \text{example : A is the base and n is the exponent. When multiplying exponents with the same. (a ) m n amn 6. X m x n = x m + n the product rule. 2^3 ⋅ 2^4 = 2^ (3+4) = 2^7 = 128. Putting all the rules together, we can simplify more complex expression containing exponents. A negative exponent means divide, because the opposite of multiplying is dividing. An am an m 4. For example, writing 4 x 4 x 4 x 4 x 4 with an exponent. A n ⋅am = an m+ 3. A n = a × a ×. The laws of exponents illustrate how to simplify numbers using the properties of. (a ) m n amn 6. For example, writing 4 x 4 x 4 x 4 x 4 with an exponent. \large\displaystyle x^m \cdot x^n = x^ {m+n} xm ⋅ xn = xm+n. Web the laws of exponents (also called rules of exponents) come from three ideas: \large \displaystyle x^0 = 1 x0 = 1, for x = \not 0. A fractional exponent like 1/n means to take the nth root: Web the product rules for exponents. \large\displaystyle x^1 = x x1 = x. An am an m 4. A n = a × a ×. $$ \boxed{ x^a \cdot x^ b = x^{a \red + b} } \\ \text{example : All numbers (not zero) raised to the zero power equal one. Web exponents rules and properties. When dividing two quantities with the same base, subtract exponents: Multiplying exponents with the same power: Web each exponent rules chart on this page summarizes how to use the power rule, fraction rule, product rule, the negative rule, log to exponents and more! \large\displaystyle x^m \cdot x^n = x^ {m+n} xm ⋅ xn = xm+n. A n an 1 to move a number or a symbol from the numerator to the denominator (or from the denominator to the numerator), you must change the sign of the exponent. Here we apply all the rules of exponents to simplify expressions. Web the exponent (the number 2) is the number of bases (the number 5) you multiply together. Web in this article, we are going to discuss the six important laws of exponents with many solved examples.Rules of Logarithms and Exponents With Worked Examples and Problems
Rules Of Exponents Chart
Laws of Exponents and Indices with Examples [Video] Teachoo
Exponent Rules Law and Example Studying math, Learning mathematics
Exponent Rules Laws of Exponents Exponent Rules Chart
Free Printable Exponent Rules Chart & Power Chart 110 [PDF
Exponent Rules Chart
Rules Of Exponents Anchor Chart
Exponents, Exponential Notation, and Scientific Notation (solutions
Web Rules Of Exponents 1.
For All Real Numbers X And Y And Real Number Constants M And N.
When Multiplying Exponents With The Same Base, Add The Powers.
When X = 1, Y = B:
Related Post:
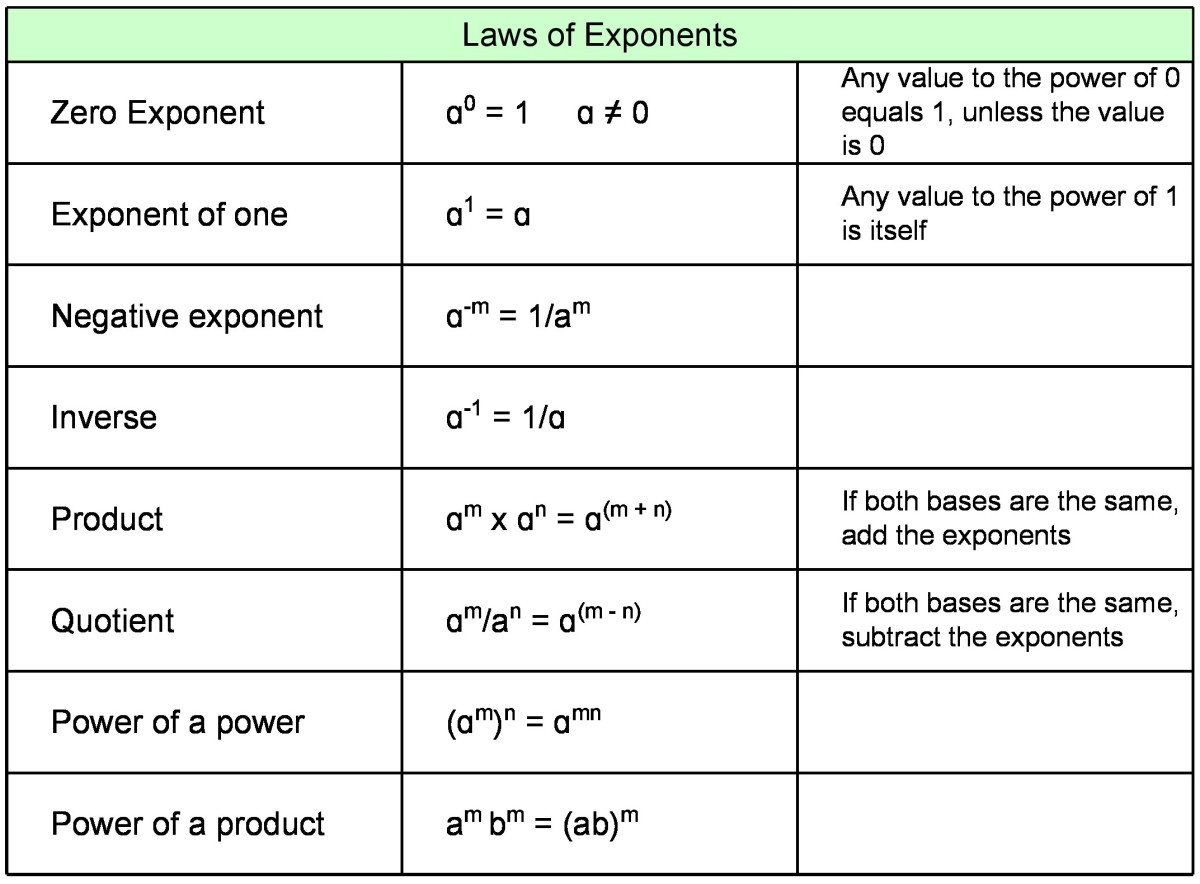

![Laws of Exponents and Indices with Examples [Video] Teachoo](https://d1avenlh0i1xmr.cloudfront.net/914d9e5c-9055-415c-8eb4-45ee41729114/exponent-law-with-examples.jpg)